Signal Denoising Application

APPLICATIONS IN SIGNAL DENOISING

In a joint work with Andrew Bruce (MathSoft, Seattle) and Sylvain Sardy (EPFL, Lausanne), we developed numerical methods for signal denoising using wavelet basis. We reformulated the problem as an optimization problem (in fact, a convex quadratic program with special structures) and we applied a block-coordinate relaxation (Gauss-Seidel) method as well as a primal-dual interior-point method to its solution. Two applications of this signal denoising problem are shown below. The images were generated by Sylvain and are taken from the technical reports detailing our work, available soon from my homepage.

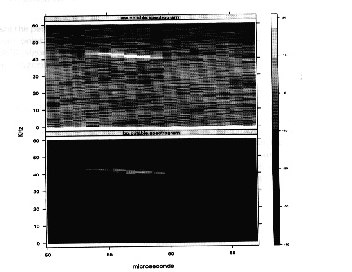
1. Electronic surveillance:

The application concerns passive detection and finger printing of incoming radar signatures from an electronic surveillance platform. The observed signal is 2048 samples from a chirped RF source at 3 decibels with jamming interference. The top image shows the observed signal and the bottom image shows the signal after it is denoised using wavelet Basis Pursuit.

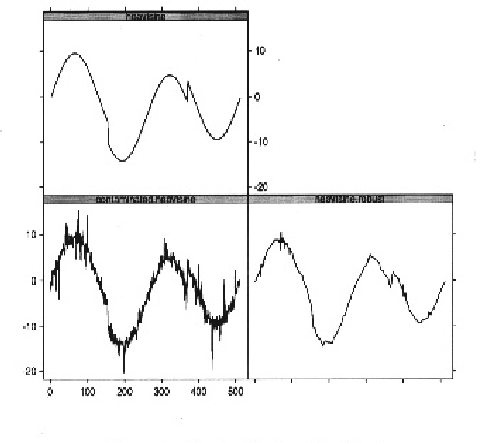
2. Denoising a contaminated function:

The top graph shows the original heavisine function. The bottom left graph shows the function after contamination. The bottom right graph shows the contaminated function after it is denoised using wavelet Robust Basis Pursuit.