MATH 318, Chapter 7 Quiz

Name:
You may use a calculator on this quiz. You may not use a cell phone or computer. Please read each question carefully, show your work and give justifications for your answers. If you find that you are spending a lot of time on one problem, leave it blank and move on to the next. If you have time left at the end of the quiz please check your work. There are questions on both sides of this quiz paper.
  1. The PharmaPlus chain of pharmacies employs 90 pharmacists and 160 technicians. As part of their "Quality Care Guarantee", PharmaPlus has pledged to employ no more than two technicians per licensed pharmacist. In addition, PharmaPlus' management has pledged to hire at least 10 new pharmacists.

    Market research predicts that the chain will need to have at least 270 employees total to meet demand in the coming year, so PharmaPlus is advertising to hire new technicians and pharmacists. Each pharmacist earns $50,000 per year and each technician earns $25,000 per year.

    Your task is to formulate a linear programming problem to help PharmaPlus determine how many technicians and how many pharmacists to hire. PharmaPlus' objective is to minimize costs while still meeting demand and satisfying the requirements of their quality care guarantee.

    You have been asked to use the following variables in your formulation:

    P = total number of pharmacists employed
    T = total number of technicians employed

    a) (10 points) What is the objective function for this linear programming problem?

     

     

     

    b) (10 points) Are you trying to maximize or minimize the value of this function? Explain your answer.

     

     

     

     

    c) (30 points) What are the constraints in this linear programming problem? Write the inequalities that describe these constraints below.

     

     

     

     

     

     

     

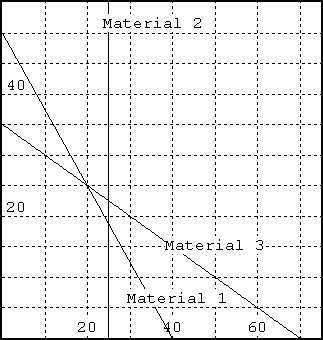
  2. RMC, Inc. manufactures two products: a solvent and a fuel additive. They require three ingredients to produce these products, which we will refer to as Material 1, Material 2 and Material 3. RMC's current stock of these raw materials is limited, so they are operating under the constraints given below; their objective is to maximize their profits.
    Maximize:25S + 40F
    Subject to:.5 S + .4 F ≤ 20 (Material 1)
    .2 S     ≤ 5 (Material 2)
    .3 S + .6 F ≤ 21 (Material 3)
    F,S≥0

    Here S represents the number of tons of solvent produced and F represents the number of tons of fuel additive. The feasible region for this linear programming problem is shown above on the right. (The intersection of the constraint lines for materials 1 and 2 is at S = 25, F = 19.)

    a) (30 points) What is the optimal solution to this problem? Circle the point corresponding to this solution on the graph above.

     

     

     

     

    b) (20 points) What materials represent the binding constraints for this problem?

     

     

Bonus: (5 points) RMC wishes to increse profits by purchasing additional materials. If they can only purchase one material, which would you recommend they purchase and why?